Allowed rotational energy level of rigid diatomic molecule
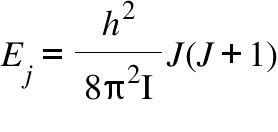
Ej = Rotational energy Level; I= Moment of Inertia; J= Rotational quantum number ; h = Plank's constant
By using Schrodinger's equation the above expression had been derived. In this expression the moment of inertia is termed as I , which is either Iy or Iz (both are same, since rotating about the y or z axis will be same for a linear molecule ) considering the linear molecule (diatomic molecule will be linear) to be aligned itself in the x direction (Ix = 0). The values are J must take integral values from zero upwards. This restriction arises due to the fact that only certain discrete rotational energy levels to the molecule is allowed.